Guided Math Part 3: Making Whole Group Instruction Work

I'm halfway through my series of posts about guided math.  Thank you to those of you who have reached out with your kind comments and questions.  I'm excited to have the chance to meet some of you in person in my upcoming seminar locations!

This week I want to focus on one of the biggest misconceptions I hear in conversations about guided math"”there isn't any whole group instruction.  I tend to disagree with this statement.  I strongly believe you can successfully implement a guided math format and keep whole group instruction.  However, as I shared in last week's post, our instruction MUST change!

Whole group instruction is generally linked with "teacher-directed" instruction.  When placing whole group instruction in a guided math classroom, the shift changes to "student-directed" instruction.  The role of the teacher is to be the facilitator and guide the instruction through rich tasks and open-ended questions, which allow for students to take control of the learning.  Students should be grouped by mixed-ability and sitting in some type of group arrangement.  This can be groups of 3, 4, 5, or even partners.

It is important that student textbooks not be present during your instruction. This is true for all grade levels.  If textbooks are open, students only need to look at the next example to answer your question.  The teacher can have the teacher's guide present to lead the instruction, but the materials you see on the pages in the book should be in your students' hands.  Instruction that is learned through the concrete, pictorial (visual), abstract continuum, is instruction that develops conceptual understanding, not just procedural understanding.

These are my tips for having a successful whole group lesson:

• Follow concrete-pictorial-abstract approach
• Students should be "doing math" during the lesson...textbooks should be closed
• ALL students should have manipulatives
• Use dry erase boards, individual chalkboards, or dry erase sleeves for students to do their recording...you can do quick formative assessment while you are teaching
• Remember numbers are abstract, always put numbers into context before students work on computation
• Include problem solving in all lessons
• Lesson is guided through teacher questioning; should be composed of more open-ended questions rather than closed questions
• Textbooks can be brought out at the end of the lesson to do a "picture walk" of what was learned throughout the lesson

Another question I am asked frequently, is how long should the whole group part of the lesson last?  In my opinion, there isn't a right or wrong amount of time.  I believe this will vary from grade level, teacher's style, and content.  Some teachers like to do this as a "mini-lesson" for about 10-15 minutes, and then continue the content in small groups during their workshop time.  For me, this format didn't work very well.  I found the content I was trying to teach took more time to cover than 10 minutes.  As I will show you in two weeks, my whole group instruction time was closer to 40 minutes.  But, during that entire time my students were grouped into small groups of mixed-ability and were expected to work together to complete different tasks.  One way to think about this, all students were engaged in small group instruction during my whole group block of time.  My role was to facilitate through each group and ask questions, check for understanding, and gather formative assessment data on each student.  As groups would work, we stopped periodically to share out thinking and ask questions.  To help in planning for these types of whole group lessons I like to share what I learned.  For students to learn math well, the following 5 components should be present in all lessons:

1. Exploration
2. Structure development (teacher led through students' exploration"”formalizing their "ideas" into the equations, methods, or procedures to be taught)
3. Journal (documentation of formal ideas)
4. Reflection (could be a "picture walk" through the textbook)
5. Practice (both guided and independent)

My favorite whole group lessons to model in classrooms come from my friend and colleague, Catherine Kuhns' books, Common Core Math in Action Grades K-2 and Common Core Math in Action Grades 3-5.

Both books have lessons that are designed to bring the math alive for your students.  They are easy to supplement with any textbook program you are using.  All standards are addressed, even if you aren't using "Common Core Standards".

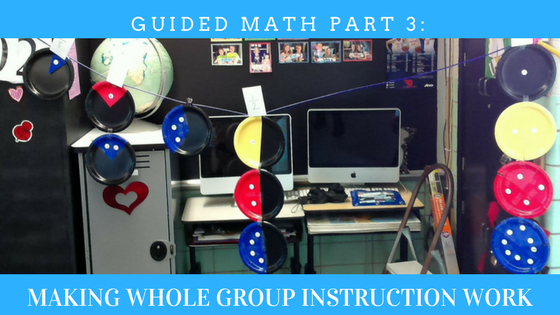
In the following pictures you see an activity by 4^th grade students who were actively engaged in learning how to place fractions on a number line using paper plates and a jump rope. 

By using concrete materials, conversation between students, connecting to a visual representation, students begin to develop the deep conceptual understanding we are hoping to achieve.  This can be developed during whole group instruction.  Next week we will look at how we connect this whole group time to our small groups.

Until next week, when our focus will be, "Small Groups With a Purpose", enjoy this week's "Plus-One":

Addition Tic-Tac-Toe

I love playing math games with kids!  This game is super easy to play with your students, requires very few materials, and your students will ask if they can keep playing!

Materials Needed for Partners:

• Addition Tic-Tac-Toe gameboard
• 2-color counters or 2 different colored Unifix cubes (about 20 altogether)
• 2 clear chips (aka Bingo chips)

Directions:

1. Partner 1 places the 2 clear chips over two addends at the bottom of the board.
2. Partner 1 adds the two numbers together and uses her color counter to place over the sum on the gameboard.
3. Partner 2 can move ONLY 1 of the clear chips at the bottom to create a new addition problem.
4. Partner 2 adds the two numbers together and uses his color counter to place over the sum on the gameboard.
5. Partner 1 continues by moving ONLY 1 clear chip to create a new addition problem and cover over the sum.
6. Play continues until one person gets 3 of their color counter in a row either horizontally, vertically, or diagonally.

Special notes:

• a student can place both clear chips over the same addend; the next player can still only move 1 of the chips to create the new problem
• if 3 in a row is too easy for your students, increase the winner to 4 in a row, 5 in a row, and so on
• allow students who still aren't fluent with their facts to use an addition table to make sure they get the correct sum